Case 24: Bespoke Fluid - Head Required for flow of 20 l/sec
Reference: 2500 Solved Problems in Fluid Mechanics and Hydraulics, 1989, McGraw-Hill, Jack B. Evett, Ph. D., Cheng Liu, M.S., Page 286, Example problem 11.17
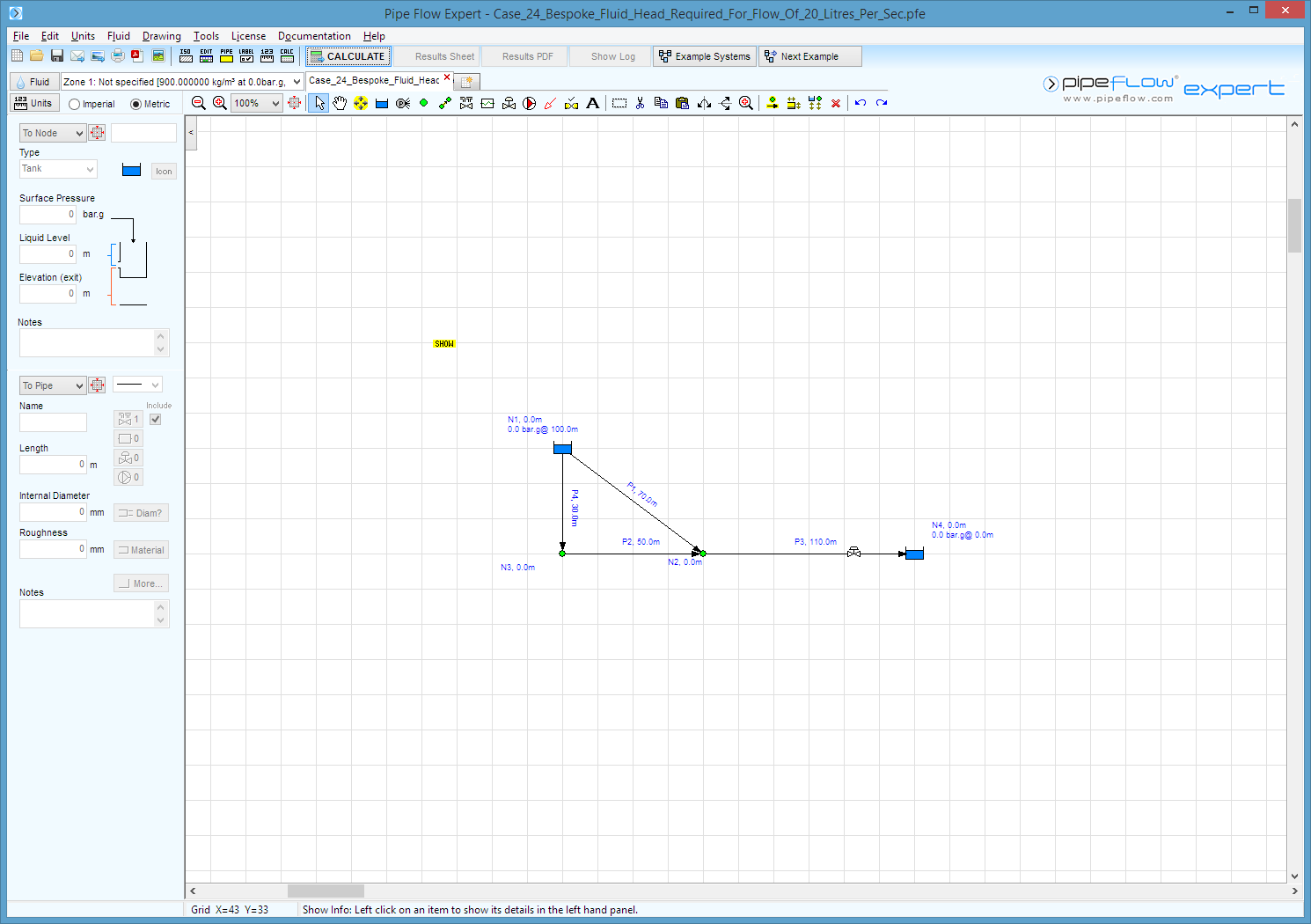
Pipe Flow Expert File: Case_24_Bespoke_Fluid_Head_Required_For_Flow_Of_20_Litres_Per_Sec.pfe
Problem Description:
A pipe system connects two tanks in which the fluid levels are different.
Two pipes connected to the tank with the highest fluid level join together at some point and the combined flow from these two pipes is carried by a third pipe towards the tank with the lowest fluid level.
The flow rate of fluid entering the tank with the lowest fluid level is 20 l/s.
Find the difference in fluid levels between the two tanks.
Fluid Data: µ = 5 x 10-3 Pa · s. Specific gravity = 0.9
Result Comparison:
|
Data Item |
Published data |
Pipe Flow Expert |
|
Difference in fluid level (m) |
49.06 |
48.757 (100 -51.243) |
|
Flow rate in pipe 1 (l/s) |
1.90 |
1.894 |
|
Flow rate in pipe 2 (l/s) |
18.0 |
18.106 |
|
Combined flow rate pipes 1 & 2 (l/s) |
19.90 |
20.000 |
Commentary:
The published data and the calculated results compare well.
The published text uses a friction factor of 0.114 read from a chart to calculate the head loss for both pipes 1 and 3.
All pipes have different diameters and different inner roughness values and carry different flow rates.
Pipe Flow Expert calculates individual friction factors for each pipe from the Colebrook-White equation.
The calculated friction factors are 0.115 (pipe 1) and 0.113 (pipe 2).
This gives a slightly different calculated head loss for each pipe, but the total fluid head difference in the system is very similar to the published text.
To model this system using Pipe Flow Expert a difference of 100 m between tank fluid levels was used.
A flow control valve was added to the pipe which carries the total flow.
The flow rate setting of this control valve was 20 l/s.
The difference in fluid levels needed to supply a flow rate of 20 l/s can be found by subtracting the pressure introduced by the flow control valve from the difference in the fluid levels. e.g. 100 m – 51.243 m = 48.757 m

