Case 55: Water – 5 Degree Bevel-edged Orifice Loss Coefficient in a Straight Pipe
Reference: Pipe Flow – A Practical and Comprehensive Guide, 2012, Publisher Wiley, Donald C. Rennels, Hobart M. Hudson, Chapter 13, Page 145
Pipe Flow Expert File: Case_55_Water_5deg_Bevel_Edged_Orifice_Straight_Pipe.pfe
Problem Description:
A straight pipe contains a 5o bevel-edged single-hole orifice. The orifice diameter to pipe diameter is specified by the ratio d0/d1. The orifice thickness to orifice diameter is specified by the ratio t/d0.
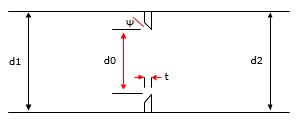
Use different orifice thickness to orifice diameter ratios (t/d0) to compare the loss coefficient (k0) through the orifice with varying orifice diameter to pipe diameter ratios (d0/d).
Pipe Flow Expert Parameters:
Fluid Data: Water at, 68 °F
One hundred and sixty-nine individual systems with inflow demands of 0.01 ft3/sec were used to model a range of bevel thickness to orifice diameter ratios (t/d0) across a range of orifice diameter to pipe diameter ratios (d0/d).
Result Comparison:
Pipe Flow Expert Calculated Results and Published Graph Readings of Orifice Loss Coefficient (k0):
|
Orifice Diameter / Pipe Diameter (d0/d) |
Orifice Thickness / Pipe Diameter (t/d0) |
Donald C. Rennels, Hobart M. Hudson (k0) |
Pipe Flow Expert (k0) |
|
0.1 |
1.0 |
2.0103 |
2.0103 |
|
0.2 |
1.0 |
1.9193 |
1.9193 |
|
0.3 |
1.0 |
1.7709 |
1.7709 |
|
0.4 |
1.0 |
1.5691 |
1.5691 |
|
0.5 |
1.0 |
1.3191 |
1.3191 |
|
0.6 |
1.0 |
1.0323 |
1.0323 |
|
0.7 |
1.0 |
0.6980 |
0.6980 |
|
0.8 |
1.0 |
0.3946 |
0.3946 |
|
0.9 |
1.0 |
0.1255 |
0.1255 |
|
0.1 |
0.5 |
2.1859 |
2.1859 |
|
0.2 |
0.5 |
2.0906 |
2.0906 |
|
0.3 |
0.5 |
1.9347 |
1.9347 |
|
0.4 |
0.5 |
1.7220 |
1.7220 |
|
0.5 |
0.5 |
1.4563 |
1.4563 |
|
0.6 |
0.5 |
1.1486 |
1.1486 |
|
0.7 |
0.5 |
0.7849 |
0.7849 |
|
0.8 |
0.5 |
0.4497 |
0.4497 |
|
0.9 |
0.5 |
0.1465 |
0.1465 |
|
0.1 |
0.3 |
2.2945 |
2.2945 |
|
0.2 |
0.3 |
2.1965 |
2.1965 |
|
0.3 |
0.3 |
2.0360 |
2.0360 |
|
0.4 |
0.3 |
1.8164 |
1.8164 |
|
0.5 |
0.3 |
1.5412 |
1.5412 |
|
0.6 |
0.3 |
1.2204 |
1.2204 |
|
0.7 |
0.3 |
0.8385 |
0.8385 |
|
0.8 |
0.3 |
0.4834 |
0.4834 |
|
0.9 |
0.3 |
0.1591 |
0.1591 |
|
0.1 |
0.2 |
2.3684 |
2.3684 |
|
0.2 |
0.2 |
2.2685 |
2.2685 |
|
0.3 |
0.2 |
2.1050 |
2.1050 |
|
0.4 |
0.2 |
1.8807 |
1.8807 |
|
0.5 |
0.2 |
1.5989 |
1.5989 |
|
0.6 |
0.2 |
1.2692 |
1.2692 |
|
0.7 |
0.2 |
0.8750 |
0.8750 |
|
0.8 |
0.2 |
0.5062 |
0.5062 |
|
0.9 |
0.2 |
0.1676 |
0.1676 |
|
0.1 |
0.15 |
2.4149 |
2.4149 |
|
0.2 |
0.15 |
2.3140 |
2.3140 |
|
0.3 |
0.15 |
2.1484 |
2.1484 |
|
0.4 |
0.15 |
1.9212 |
1.9212 |
|
0.5 |
0.15 |
1.6352 |
1.6352 |
|
0.6 |
0.15 |
1.3000 |
1.3000 |
|
0.7 |
0.15 |
0.8979 |
0.8979 |
|
0.8 |
0.15 |
0.5206 |
0.5206 |
|
0.9 |
0.15 |
0.1728 |
0.1728 |
|
0.1 |
0.1 |
2.4732 |
2.4732 |
|
0.2 |
0.1 |
2.3708 |
2.3708 |
|
0.3 |
0.1 |
2.2028 |
2.2028 |
|
0.4 |
0.1 |
1.9720 |
1.9720 |
|
0.5 |
0.1 |
1.6808 |
1.6808 |
|
0.6 |
0.1 |
1.3385 |
1.3385 |
|
0.7 |
0.1 |
0.9265 |
0.9265 |
|
0.8 |
0.1 |
0.5384 |
0.5384 |
|
0.9 |
0.1 |
0.1793 |
0.1793 |
|
0.1 |
0.07 |
2.5181 |
2.5181 |
|
0.2 |
0.07 |
2.4146 |
2.4146 |
|
0.3 |
0.07 |
2.2447 |
2.2447 |
|
0.4 |
0.07 |
2.0110 |
2.0110 |
|
0.5 |
0.07 |
1.7158 |
1.7158 |
|
0.6 |
0.07 |
1.3681 |
1.3681 |
|
0.7 |
0.07 |
0.9485 |
0.9485 |
|
0.8 |
0.07 |
0.5522 |
0.5522 |
|
0.9 |
0.07 |
0.1842 |
0.1842 |
|
0.1 |
0.05 |
2.6037 |
2.6037 |
|
0.2 |
0.05 |
2.4980 |
2.4980 |
|
0.3 |
0.05 |
2.3245 |
2.3245 |
|
0.4 |
0.05 |
2.0854 |
2.0854 |
|
0.5 |
0.05 |
1.7826 |
1.7826 |
|
0.6 |
0.05 |
1.4245 |
1.4245 |
|
0.7 |
0.05 |
0.9904 |
0.9904 |
|
0.8 |
0.05 |
0.5782 |
0.5782 |
|
0.9 |
0.05 |
0.1935 |
0.1935 |
|
0.1 |
0.03 |
2.6354 |
2.6354 |
|
0.2 |
0.03 |
2.5290 |
2.5290 |
|
0.3 |
0.03 |
2.3541 |
2.3541 |
|
0.4 |
0.03 |
2.1130 |
2.1130 |
|
0.5 |
0.03 |
1.8073 |
1.8073 |
|
0.6 |
0.03 |
1.4455 |
1.4455 |
|
0.7 |
0.03 |
1.0060 |
1.0060 |
|
0.8 |
0.03 |
0.5878 |
0.5878 |
|
0.9 |
0.03 |
0.1969 |
0.1969 |
|
0.1 |
0.02 |
2.7088 |
2.7088 |
|
0.2 |
0.02 |
2.6006 |
2.6006 |
|
0.3 |
0.02 |
2.4226 |
2.4226 |
|
0.4 |
0.02 |
2.1769 |
2.1769 |
|
0.5 |
0.02 |
1.8646 |
1.8646 |
|
0.6 |
0.02 |
1.4939 |
1.4939 |
|
0.7 |
0.02 |
1.0419 |
1.0419 |
|
0.8 |
0.02 |
0.6101 |
0.6101 |
|
0.9 |
0.02 |
0.2048 |
0.2048 |
|
0.1 |
0.01 |
2.7492 |
2.7492 |
|
0.2 |
0.01 |
2.6400 |
2.6400 |
|
0.3 |
0.01 |
2.4603 |
2.4603 |
|
0.4 |
0.01 |
2.2120 |
2.2120 |
|
0.5 |
0.01 |
1.8962 |
1.8962 |
|
0.6 |
0.01 |
1.5206 |
1.5206 |
|
0.7 |
0.01 |
1.0617 |
1.0617 |
|
0.8 |
0.01 |
0.6224 |
0.6224 |
|
0.9 |
0.01 |
0.2091 |
0.2091 |
|
0.1 |
0.005 |
2.5550 |
2.5550 |
|
0.2 |
0.005 |
2.4510 |
2.4510 |
|
0.3 |
0.005 |
2.2795 |
2.2795 |
|
0.4 |
0.005 |
2.0435 |
2.0435 |
|
0.5 |
0.005 |
1.7449 |
1.7449 |
|
0.6 |
0.005 |
1.3927 |
1.3927 |
|
0.7 |
0.005 |
0.9668 |
0.9668 |
|
0.8 |
0.005 |
0.5635 |
0.5635 |
|
0.9 |
0.005 |
0.1883 |
0.1883 |
|
0.1 |
0.001 |
2.6781 |
2.6781 |
|
0.2 |
0.001 |
2.5706 |
2.5706 |
|
0.3 |
0.001 |
2.3939 |
2.3939 |
|
0.4 |
0.001 |
2.1501 |
2.1501 |
|
0.5 |
0.001 |
1.8406 |
1.8406 |
|
0.6 |
0.001 |
1.4736 |
1.4736 |
|
0.7 |
0.001 |
1.0269 |
1.0269 |
|
0.8 |
0.001 |
0.6008 |
0.6008 |
|
0.9 |
0.001 |
0.2015 |
0.2015 |
Graphical Comparison of Formula:
Commentary:
The published loss coefficients compare well with the calculated results.
Bevel Limit: There is a point where beveling is limited by the available radial distance between the pipe wall and the face of the orifice. For a 50 bevel angle this limitation occurs when d0/d is greater than about 0.87 and hence this is not significant when considering the points on the above graph.
Note: Head Loss in m fluid = (k0 * v2) / 2g
- where v = fluid velocity in m/s at the entrance to the orifice, g = acceleration due to gravity in m/s2
- k0 is not the same as a standard k value (which is used in formulas where v = velocity in the pipe)

